
Near Doubles Strategy
The near doubles strategy helps make addition easier for first and second graders. This method is used when two numbers are close together like 7 + 8. Students use their knowledge of the doubles math strategy to help them solve other facts. This builds their math fact fluency, their number sense, and their flexibility with numbers. This strategy is sometimes also called the doubles plus one strategy or math near doubles.
The near doubles math strategy is one that students can use when building their math fact fluency through playing games. Students need many opportunities to practice building their fluency and games are a perfect way to do this! They make it fun and engaging, and students forget that they are practicing their math facts. Use this Dot Addition or Dot Subtraction in your classroom. Put your name and email address in the boxes, and it will be in your inbox. It is easy to prep, and your students will love it!
What Are Near Doubles in Math?
A near doubles fact involves adding two numbers that are almost the same such as 7 + 8, 5 + 6, or 9 + 10. To solve a near doubles fact, students can start with a doubles fact they already know. When I begin teaching the doubles plus one strategy, I always begin by having them double the smaller number. Since we have already learned our doubles facts, this makes it easy to move to the near doubles strategy.

Here are some doubles plus one examples.
7 + 7 = 14 (a doubles fact they know)
7 + 8 is just one more than 7 + 7 so we add one more to 14 which makes 7 + 8 = 15.
5+5=10 (another doubles fact they know)
5+6 is just one more than 5+5 so we add one more to 11 which makes 5+6=11.
Step 1: Modeling with Manipulatives for the Near Doubles Strategy
One of the best ways to teach the near doubles strategy is through hands-on practice with manipulatives. This gives students a concrete visual to help them understand the relationship between the doubles fact and the near doubles fact.
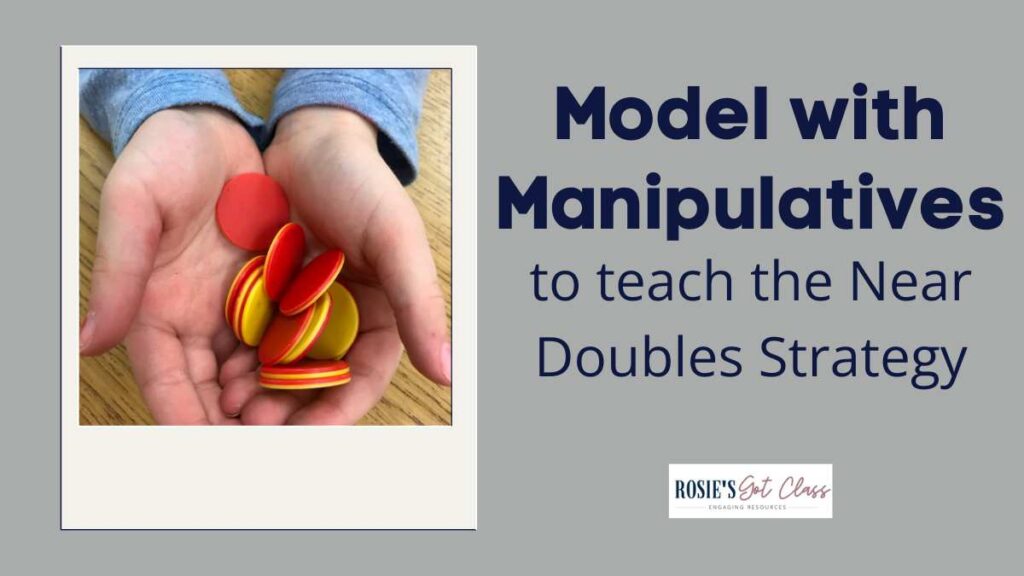
Start by using ten frames and two different colors of manipulatives (like unifix cubes or two-colored counters). Here’s how I break it down:
1. Model the doubles fact using the smaller number first. For example, if you’re working on 7 + 8, begin by showing 7 + 7 using one color. Fill in both ten frames with seven manipulatives of the same color.
2. Then model the near doubles fact. Explain that when we have 7 + 8, it’s just one more than 7 + 7. Add one more manipulative to the ten frame in a different color. This helps students see visually that 7 + 8 is just one more than 7 + 7.
3. Repeat the process with different examples such as 6 + 7 and 8 + 9. Make sure you always begin with the smallest doubles fact first and then add one more to find the near doubles total.
Step 2: Practice with Manipulatives
Once you’ve modeled a few examples, it’s time for students to use the manipulatives themselves. Give each student two ten frames and a set of manipulatives in two different colors. Small erasers or two-colored counters work great for this.
1. Have the students model a doubles fact, such as 6 + 6. They should place the manipulatives on the ten frames and should all be in the same color.
2. Next, ask them to model the near doubles fact. For 6 + 7, they will add one more manipulative in the second color. This will help them visually see that 6 + 7 is just one more than 6 + 6.
3. Continue practicing with other examples and allow the students to model both the doubles and near doubles facts with their manipulatives.

This step reinforces the concept while keeping it fun. By using manipulatives, they help students stay engaged and make the learning experience more memorable as they practice the near doubles strategy.
Step 3: Drawing Ten Frames and Writing Equations
After practicing with manipulatives, students can begin transitioning to more abstract representations of the near doubles math strategy. This involves drawing in ten frames and writing equations.
1. Have the students draw a doubles fact in two ten frames. I usually have them draw circles because they can be drawn quickly. I have them draw the doubles fact in one color. For example, I would have them draw 6 circles in one ten frame and 6 circles in another ten frame using a red crayon for the doubles fact of 6 + 6. Then I would have them draw one more circle with a blue crayon color to show the near doubles fact of 6 + 7.
2. After drawing using the ten frames, I guide them in writing out the equations that match what they’ve drawn. For example:
6 + 6 = 12
6 + 7 = 13 (by adding one more to 12)
This combination of drawing and writing reinforces the concept and helps students internalize the strategy.
Second Method: Doubling the Bigger Number with the Near Doubles Strategy
Once students are comfortable with doubling the smaller number and adding one, I introduce the concept of doubling the bigger number and then subtracting one.
For example, with 7 + 8, 8 is larger so we double it. Then we discuss if we should add one because that is what we have been doing. I love to hear their thoughts and explanations. You can really see who has good number sense with this discussion. It doesn’t take long before we decide that we need to subtract one because we doubled the bigger number. Then we subtract one to get the answer: 16 – 1 = 15.
This method works just as well and helps students develop flexibility with numbers. I practice this same strategy with the class by first using manipulatives, letting students practice, and finally having them draw and write equations.
Choosing the Best Method
After practicing both methods, I talk to students about choosing the strategy that works best for them. Some students may find it easier to double the smaller number and add one while others might prefer doubling the bigger number and subtracting one. I also mention that they might want to use the easiest doubles fact for them—whether it’s the smaller or bigger number.
Making It Fun
To keep this activity enjoyable, use engaging manipulatives like unifix cubes, small erasers, or two-colored counters. Children like to have fun. When they do, they are more likely to internalize the strategy.

In conclusion, the near doubles strategy is an excellent tool for first and second graders learning to add. By starting with a doubles fact and adjusting by one, students can solve addition problems with confidence. Through hands-on practice with manipulatives, drawing, and writing equations, students will solidify their understanding of this strategy and build their number sense.
You might also enjoy these articles.
Near Doubles Addition Strategy
Using Doubles Strategy for Addition
Near Doubles Second Grade Addition Facts


